Infinitesimal and
infinity concepts in mathematics amaze me. Some unorganized thoughts:
How can we prove that dx^2=0? Is the following reasoning correct (thanx to Fatih):
Let "x" be a real number in the interval (0,1). We know that in that interval x^2 is smaller than x. Now dx is smaller than 1. Then, dx^2 must be smaller than dx. But since dx is an infinitesimal number, the only number smaller than dx is zero.
Why does an infinitesimal rotation not change the orientation of a vector? This assumption is employed in finding the derivative of a unit vector in polar coordinates:
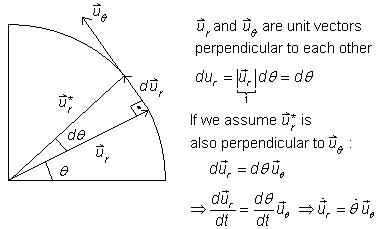
My question on wikipedia's
infinitesimal talk page.
How can we know that the usual arithmetic operations like +, -, *, / are valid or invalid for infinitesimal and infinite numbers?
What does "undefined" mean in mathematics and how do we claim that 0/0, inf/inf, inf-inf, inf/0 are undefined? Can we use the following argument:
1/inf = 0 = 2/inf. But we can't do this: (1/inf)*inf=(2/inf)*inf which leads to the contradiction 1=2. Is that the reason we say inf/inf is undefined?
What's the difference between zero and void (nothingness)?
2 apples - 2 apples = zero
or
2 apples - 2 apples = void?
"No single mathematical creation has been more potent for the general on-go of intelligence and power." G.B. Halsted, referring to zero





3 comments:
0/0 uzerine yorumlar:
1) 0/0'i limitler uzerinden yorumlandiginda dikkat ceken husus limitteki 0/0 degerinin origine (x,y)=(0,0) nasil yaklasildigina bagli oldugudur.Ornegin x/y ifadesi x ve y sifira giderken aldigi deger (x(p),y(p)) egrisinin egimine bagli cikmaktadir (bu noktada L'Hospital kuralini hatirlamkata fayda var). Eger x,y theta egimli cizgiler uzerinde origine yaklasiyorsa x/y=cotan(theta) limit degerine varacaktir.
2) 0/0 Boole'nin kitabinda rastladigim "indefinite" yorumuna bakmakta fayda olabilir. Orda 0/0'in insan aklinin isleyisini tarif eden cebirde (Yani kitap boyunca anlatilan "Boolean Algebra") yorumu almakta (. Guzel haber ki Boole'nin kitabi artik Gutenberg project altinda yer aliyor (http://www.gutenberg.org/etext/15114) (sayfa 44. sembolun tanitilmasi, sayfa 73 normal algebra ile iliskisi)
"An Investigation to the Laws of Thought" pdf'ini indirdim.
s.44'te anlatılanların mevzu ile ilgisini anlamadım. Aynı şekilde s.73'te de birşey yok. Aceba farklı versiyonlara mı bakıyoruz?
s44. te tanitilan sembol v, 0/0 yerine "indefinite" anlaminda kullaniliyor. Ikinci sayfayi yanlis vermisim 56-66 olacak, ordada bahis devam ediyor. Bu sayfalarda vurgulanan fikirlerden biri normal cebir ile Boole'nin gelistirdigi cebir arasindaki benzerlikler. Buna gore herhangi bir "proposition" "expand" edildigi zaman "constituent" katsayilari 0/0 oldugunda "indefinite" (belirsiz => hepsi de olabilir, hic te, bir kismi da), 1/0 oldugunda ise evrensel set olarak (bu durumda "constituent" ifadeden cikarilip tek basina birakilmaktadir zira sol taraf sonsuzlugu temsil etmediginden sag tarafta boyle bir terim olmasi mumkun degildir) kabul edilmekte. Sonucta "proposition" analiz edildiginde sonsuzluk , hiclik ve belirsizlik olarak yorumlanan rakamlar beliriyor analiz bunun uzerinden devam ediyor. Sonucta kitap senin mevzuna direk ilac olmamakla beraber, analojiyi yakaladiginda yardimci olabilir.
Post a Comment